1.Summer vacations in a school started from a particular Monday in the month of April. The last day in the month of May of the same year was Wednesday. If the summer vacations started from the third Monday of April, then on which date did the summer vacations start?
a) 18th April.
b) 17th April.
c) 16th April.
d) 15th April.
May has 31 days.
If the last day of May was Wednesday, then 3rd May was also Wednesday.
This implies that 30th April was Sunday.
Therefore, 24th April was Monday and 17th April was a Monday.
Since, the vacations started from the third Monday of April, we can conclude that the summer vacations started on 17th April.
2.

Source: safalta
How many non-null subsets does the set of odd composite numbers between 1 and 100 have?a) (225 – 1). b) (224 – 1). c) 224 d) 225
There are 50 odd numbers between 1 and 100.
Out of which 1 is neither prime nor composite.
Similarly, out of 25 prime numbers between 1 and 100, one prime number (2) is even and remaining 24 are odd.
Therefore the number of odd composite numbers between 1 and 100 is : 50 – 1 – 24 = 25
Number of non-zero subsets of a set with ‘n’ elements = 2n – 1
Therefore, required answer is (225 – 1).
3. The power consumed by an air conditioner is partly fixed and partly varies with the number of hours for which the air conditioner is switched on. The ratio of the power consumed when the air conditioner is switched on for 4 hours and 16 hours is 1 : 3. What will be the ratio of power consumed when the air conditioner is switched on for 6 hours and 10 hours?
a) 2 : 5 b) 2 : 3 c) 1 : 3 d) 1 : 2
Let the power consumed, fixed component and variable component be P, x and y respectively.
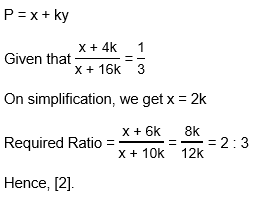
4. Election was held to select the chairman of the governing council of ICC. The result of the election indicated that 25% of the people voted for Ravi and the rest voted for Sunil. Later it was discovered that the voting machine was faulty and 20% of the people whose votes were recorded in favour of Ravi had actually voted for Sunil and 50% of the people whose votes were recorded in favour of Sunil had actually voted for Ravi. If the difference between the people who actually voted for Ravi and those who actually voted for Sunil was 3,00,000, how many people actually voted for Ravi?
a) 20,00,000 b) 12,50,000 c) 16,80,000 d) 11,50,000
Let the total number of people who voted be x.
Votes recorded for Ravi = 0.25x and votes recorded for Sunil = 0.75x
People who actually voted for Ravi = 0.25x – 0.2(0.25x) + 0.5(0.75x) = 0.575x
People who actually voted for Sunil = 0.75x – 0.5(0.75x) + 0.2(0.25x) = 0.425x
Difference = 0.575x – 0.425x = 0.15x = 300000
x = 20,00,000
Number of people who actually voted for Ravi = 0.575(2000000) = 11,50,000
Hence, [4].
5.
Two identical circles are as shown below.
Find the area of the shaded region.
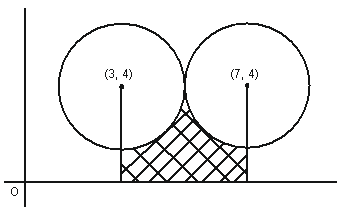
a) 16 - 2π b) 32 - 4π c) 16 - π d) 16 - 3π

6. If the roots of the equation (x – a)(x – 2b) = 6 are 0 and –1, then what is the sum of the roots of the equation (x + 2a)(x + 4b) = 0?
a) – 2 b) 1 c) 2 d) -1
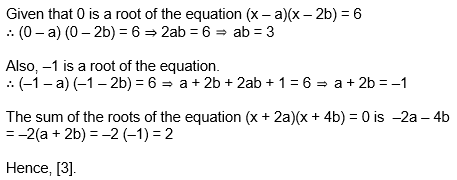
7. Given that two roots of 2x3+ ax + b = 0 are 5 and −4. What is the value of (a + b)?
a) -84 b) -82 c) -42 d) -32
2(5)3 + a(5) + b = 2(–4)3 + a(–4) + b
250 + 5a + b = –128 – 4a + b
Solving this, we get a = –42
2x3 + ax + b = 0
Substituting value of a = -42 and x = -5, we get
250 – 210 + b = 0
b = –40
a + b = (–42) + (–40) = –82
Hence, [2].
8. A tank has an inlet pipe attached to it. The inlet pipe can fill the tank such that the tank could be completely filled at 10 AM if it is opened in the morning of the same day. However, the pipe had a leak, which was not noticed. As a result of the leak, the tank was only 60% full at 10 AM. What was the ratio of the rate at which the inlet pipe fills the tank to that at which the leak empties the tank?
a) 5 : 2 b) 7 : 3 c) 2 : 1 d) 8 : 3
In the absence of leak, the tank would have been 100% full at 10 AM.
That means the leak empties 40% of the tank till 10 AM.
The ratio of the rate at which the pipe fills the tank to the rate at which the leak empties the tank = 100 : 40 or 5 : 2
Hence, [1].
9.
a) 41/3 b) 31/3 c) 21/3 d) 2
10.
The nth term of an AP is 48 and the (n + 10)th term of the same AP is 88.
Find the sum of all the terms of the AP from 48 to 88 (both included).
a) 748
b) 792
c) 802
d) 776




